An application of metric geometry to robotics
My dissertation concerned an application of metric geometry to the problem of reconfiguring robotic systems.
I’m providing it here for any of my students who may be curious what doctoral-level math looks like—or what I was talking about in class that time I was saying how moving a robot arm with two 360° joints is like drawing a curve on a donut.
- If you’re viewing this in a browser, use the slideshow presentation WITHOUT animations.
- Here’s a version of the slideshow WITH animations. Don’t view it in a browser.
- The animations will play in Adobe Reader if you click on the first broken movie icon you see and choose “Trust this document”:
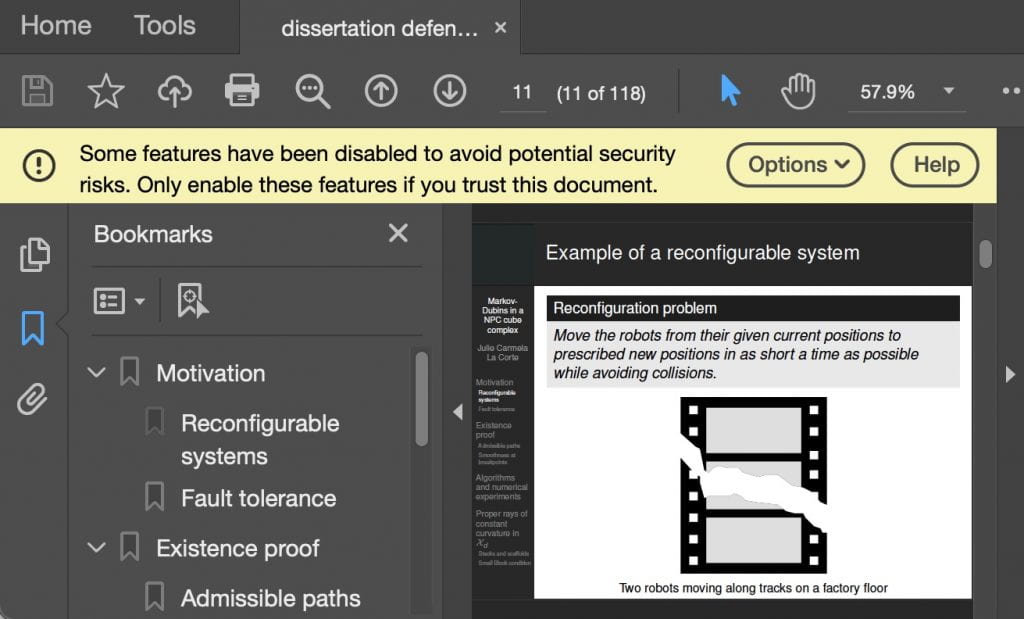
- The human-readability of the dissertation itself—“The Markov-Dubins problem with free terminal direction in a nonpositively curved cube complex”—can be guessed at based on the title. Some of the pictures (starting at p. 95, say) are pretty neat, though.
Leave a Reply